BiSON consists of a network of six remote solar observatories monitoring low-degree solar oscillation modes. It is operated by the High Resolution Optical Spectroscopy group of the School of Physics and Astronomy at the University of Birmingham, UK. We are funded by the Science and Technology Facilities Council (STFC).
A Journey to the Center of the Sun
Introduction
Helioseismology is the study the interior of the Sun by observation of its natural resonant modes of oscillation. In summary, the Sun resonates like a wind instrument, with the dominant periods observed at about five minutes. The Sun acts as a natural cavity to trap sound, which is generated by turbulence in the outermost few-hundred-kilometers of the convection zone. While this thin ‘generation’ layer occupies but an insignificant fraction, by radius, of the entire star, the sound made within the layer can in principle penetrate to the very centre of the Sun.
Standing waves set up in three dimensions give acoustic modes, which provide a probe of the interior layers that the constituent waves traverse. We specialise in the observation and study of modes formed by waves that penetrate the core of the Sun. Without these modes, scientists would not be able to map out the structure of the deep interior of the star.
Through helioseismology, the Sun fulfils the role of a Rosetta stone for astrophysics. Helioseismology allows us to study the Sun in great detail. Through this we can inform our understanding of the life histories of the Sun and stars. Stars are, after all, one of the structural ‘building blocks’ of the universe, and the keys to supporting life. Helioseismology also allows us to test fundamental physics, under the exotic conditions found in the solar interior. Perhaps the most conspicuous example of this was the use of helioseismology to ‘test’ the solar neutrino problem, which pointed the way to a solution in particle physics.
The analysis techniques that we apply to `Sun-as-a-star’ data are also applicable to observations of oscillations of distant, Sun-like stars: asteroseismology. Results from asteroseismology will test stellar evolution theory even more stringently, i.e., can theories predict the structures of a selection of stars at different points on their life cycles? It will also provide crucial, further information for stellar dynamo modellers, who seek to understand the origins of activity on stars.
Solar Oscillations
Properties of sound waves in the Sun
In 1970 Roger Ulrich at UCLA suggested that sound waves travelling from the interior of the Sun up to the surface would be reflected back in again at the surface boundary. Imagine a wave normal to the surface of the Sun and travelling towards the centre. As the wave travels deeper into the interior, the temperature increases and the wave is progressively refracted away from the normal until it turns around and returns to the surface. At the Sun’s surface the sharp density gradient causes subsequent reflection and the wave heads back into the Sun.
The more often a wave returns to the surface, the less deeply it penetrates before being turned back (the green path). Conversely, waves reflected only a few times from the surface probe much deeper into the Sun (the blue path).
Just as with conventional sound waves in a musical instrument, sound waves in the Sun can constructively reinforce to produce a standing wave. The constructive and destructive interference of the sound waves result in regions of low and high pressure within the Sun’s interior. As the Sun is a three dimensional object, these pressure nodes are distributed throughout its interior and solar scientists use a numbering convention to describe the number of nodes present in a particular standing wave configuration. The letters n, l and m are used to represent the number of nodes distributed throughout the spherical geometry of the sun where:
- n is the number of nodes radially outward from the centre of the Sun
- m is the number of nodes found around the equator
- l is the number of nodes found around the azimuth (a great circle through the poles)
- m can take any value from -l to 1. A particular arrangement of n, m, and l is known as a mode of oscillation. For example, by using this notation stellar physicists can distinguish between the n = 20, l = 2, m = 2 and the n = 20, l = 1, m = 1 modes.
The following diagram illustrates various modes of oscillation of the Sun with their corresponding values of n, l and m.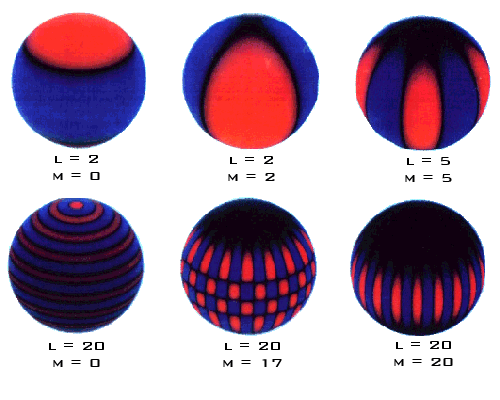
In the diagrams to the left you can think of the blue regions as moving towards you and the red regions moving away from you. At HiROS we measure the Doppler shift from such regions. Information from the whole surface of the Sun is collected and then interpreted to find information about the standing waves in the Sun’s interior which cause it to oscillate. Because some of these modes are formed by sound waves which penetrate into the deep interior of the Sun, this provides scientists with an unprecedented opportunity to study these core regions, where the nuclear reactions which power the Sun and also drive its evolution (and, of course, provide us with a comfortable environment here on Earth!) take place. These core-penetrating modes were first observed by the HiROS group in the mid-1970’s. As with a musical note produced in a flute, say, there will be a fundamental mode of vibration with higher harmonics imposed on top. By using Fourier analysis, specifically the use of the Fourier transform, it is possible to find the frequencies of the notes that make up the total signal. These techniques are applied to the modes of vibration of the sun to obtain the frequencies present in the oscillations.